The Return of Common Core Math

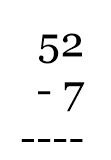
Last year, I wrote about how NHL went into a full blown panic over his math homework.  I featured one of his problems and compared the Common Core method of solving it against the "Old School" method.  I concluded at the time that not only was the Common Core method more work than was necessary (drawing pictures instead of actually dealing with the numbers), but its methodology wouldn’t scale to larger problems.  I haven’t seen much of NHL’s math work this year, mainly because he finishes it all in school.  I managed to steal a glance at his homework tonight and it actually seems sane.

JSL, on the other hand, actually shed tears thanks to his homework recently.  After helping him with it for a few days, I figured I’d do another Common Core vs. Old School Math comparison.

Let’s start with a random problem from JSL’s most recent homework.  What is 52 – 7?

We’ll start with the Old School method.  First, we display the problem like so.

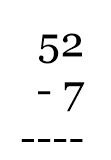
Next, we try to subtract 7 from 2.  Seeing this isn’t possible, we take one off of the 5 and add 10 to the 2.

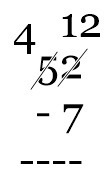
Now, we can subtract 7 from 12 and get 5.

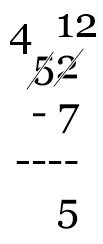
Finally, we subtract in the tens place – easy since it is 0 from 4.

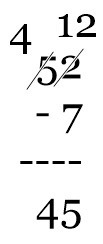
And we have our answer: 45.  Not hard at all, right?

 

Now, let’s see how the Common Core method does it.  First you write out the problem.

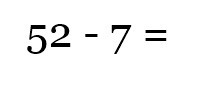
So far, so good.  Now you break 10 off of the 52 like so:

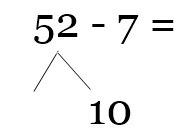
Taking 10 off of 52 leaves 42 left so we’ll fill that in too.

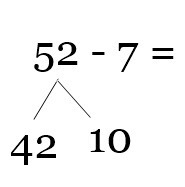
Now we subtract the 7 from the 10 and get 3.

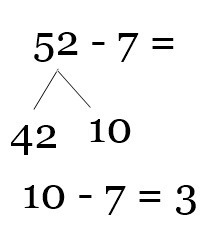
Finally, we add that 3 to the 42 to get 45.

So we have our answer: 45.

Looking at both methods, I understand what the Common Core authors were shooting for.  They were trying to take the "borrow 10" step from Old School math and recreate it in a more visual fashion.  The problem I have with this is that it needlessly complicates the math problem.  52 – 7 turns into three separate problems: 52 – 10 = 42, 10 – 7 = 3, and 42 + 3 = 45.  There’s also the unwritten math problem of what tens break out do you need to do. After all, if the problem was 52 – 16, breaking 10 out wouldn’t help.  Instead, you would need to pull 20 out of 52 and adjust the rest of the problem accordingly.

In the end, the Common Core method isn’t horrible.  Certainly, not as bad as last year’s math.  Still, it is needlessly complex and not developmentally appropriate for a second grader.  I’m all for progress, but everything new isn’t necessarily better.  In this case, the Old School method is easier and more direct while the Common Core method is more confusing.  The good news is that I think my son has finally figured out how to do this math.  The bad news is that he shouldn’t have had to.